On the n-th decimal digit of pi
Define the integer-valued function ![]() to return the
to return the ![]() -th decimal digit of
-th decimal digit of ![]() . This is the sequence A000796 in the OEIS.
. This is the sequence A000796 in the OEIS.
We will now state a conjecture that is inspired by a limit appearing in an elementary proof of Stirling’s approximation by Jakub Smolík [1]. In his proof, Smolík shows:
![]()
Conjecture. Let ![]() such that
such that ![]() . Then, the
. Then, the ![]() -th decimal digit of
-th decimal digit of ![]() , denoted as
, denoted as ![]() , is given by the expression:
, is given by the expression:
![]()
We offer a partial proof of this conjecture:
Considering the term within the floor function, we can rewrite it as
![]()
We observe that
![]()
So
![]()
Thus, by choosing ![]() sufficiently large, we can extract the
sufficiently large, we can extract the ![]() -th decimal digit of
-th decimal digit of ![]() as
as
![]()
It remains only to prove that ![]() is large enough to ensure that the formula holds for all
is large enough to ensure that the formula holds for all ![]() .
.
An arithmetic term is a fixed-length elementary closed form expression, applicable to all ![]() , which uses only the operations of addition, multiplication, bounded subtraction, division with remainder, and exponentiation.
, which uses only the operations of addition, multiplication, bounded subtraction, division with remainder, and exponentiation.
Our representation of the ![]() -th decimal digit of
-th decimal digit of ![]() constitutes an arithmetic term. The fact that our formula contains factorials may seem to rule this out, however,
constitutes an arithmetic term. The fact that our formula contains factorials may seem to rule this out, however,
we can rewrite our formula for ![]() using the arithmetic term for factorials below:
using the arithmetic term for factorials below:
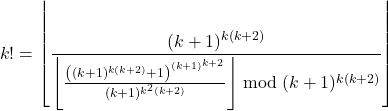
The above factorial formula is derived from results of Julia Robinson [2], who first showed that
![]()
and for ![]() :
:
![]()
References
[1] J. Smolík. “An Elementary Proof of Stirling’s Formula.” arXiv preprint arXiv:2310.04872. URL https://arxiv.org/pdf/2310.04872 (2023).
[2] J. Robinson. “Existential definability in arithmetic.” Transactions of the American Mathematical Society (1952).